

John Kelley and Gary Hill, UW-Madison, 3 July 2007
The MAM (muon absorption model) analysis, originally developed by Hill et al., compares the hit timing residuals of downgoing muons to those of Monte Carlo and uses the slope differences as a function of depth to correct the absorption of the MC ice model to the data.
We have performed the analysis using three different Monte Carlo ice models (PTD, Millennium, and Elves) and, comparing to 2005 data, have constructed an average absorption as a function of depth (see our talk from Lake Geneva). The absorption shows larger peaks and troughs in the dust and clean layers than the Millennium ice model. Finally, we compare our independent result to the new AHA ice model by Johan Lundberg and Kurt Woschnagg.
We find significantly better agreement between our result and AHA than with Millennium, supporting the conclusion that the ice absorption structure in Millennium is "smeared out" (see the wiki above on AHA for more information) and providing independent support for AHA.
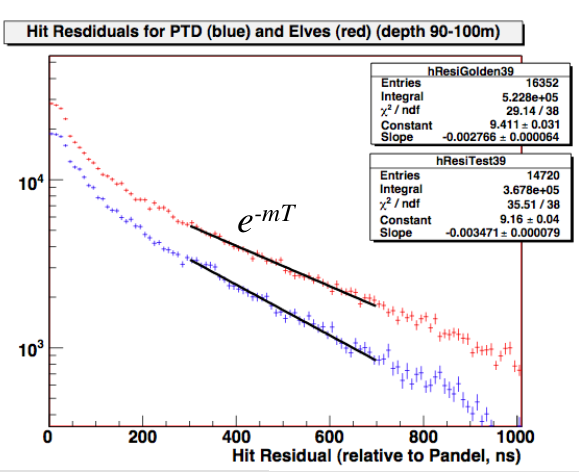
In the diffusive regime, hit timing residuals from a muon track follow an exponential decay (see Figure 1). The value of the slope depends both on the scattering and absorption of the ice (but more strongly on the latter). With the ansatz that differences between MC and data timing residuals are due to differences in absorption, one can use the slope difference to correct the MC absorption to the data.
 |
 |
Figure 1: Left — hit residuals relative
to Pandel reconstruction for two MC ice models.
Right — difference between residual slopes in this regime between data
and Millennium ice.
This correction takes the simple form:

where lambda is the absorption length, m is the residual slope, and n is the index of refraction. For lambda_MC we take the peak absorption length (as a function of wavelength).
Before we analyze the data, we can use a given MC ice model as our target unknown ice, and then compare MC-MC residual slopes to see if we can reconstruct the target ice. If the slope differences between the target and the test model are moderate to large, the procedure works reasonably well:

Figure 2: MC-MC test reconstructing PTD ice properties using
Millennium as a test model.
Corrected Millennium (red) follows PTD fairly well, especially in high absorption
regions.
If the ice models are close, though, the slope differences are too small to faithfully reconstruct the input. Fortunately (in a sense), as can be seen in Figure 1, the differences between simulation and the data are quite large, giving us reason to believe we can accurately reconstruct the true absorption in the ice.
MC simulation was performed with both PTD and Photonics, using dcorsika 6.5 + SIBYLL. About 5 million downgoing events, with a harder E^-1.7 spectrum, were generated for each ice model. A slimmed-down version of the 2005 filtering chain was applied to the MC, as well as to 0.774 days of 2005 data.
The following quality cuts were applied to ensure the timing residuals from the reconstructed tracks were meaningful:
Various different sets of cut parameters were explored, but these (same as the original MAM cuts) turned out to be a good balance between quality of the tracks and keeping enough statistics to perform the analysis.
A few systematic effects were found while investigating this method. The first is that Photonics residuals (and presumably data) are actually probing the ice about 30m below the depth of the OM (see Figure 3). This effect, as one would expect, does not show up in PTD, as photons do not cross ice layers in that simulation framework. This depth shift is applied when deriving the final absorption model.
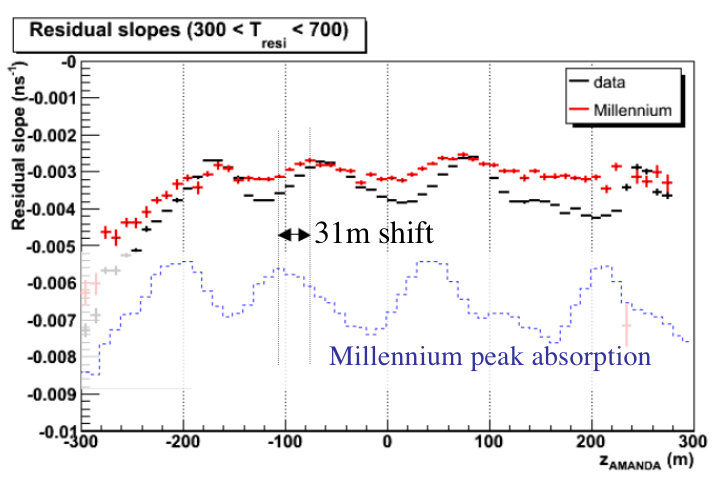
Figure 3: Depth shift between residual slopes and dust layers.
Residuals from Photonics, and presumably data, are probing the ice ~30m below
the OM.
The analysis results presented at Lake Geneva used one additional cut: using the residuals only from the first hit. Further analysis has shown this to be the cause of the overall shift to higher absorption presented in those results. If one uses all the hit residuals, this shift disappears. Results shown below use the latter approach.
The analysis has been applied to three different MC sets, each compared to data and used to estimate the actual absorption in the ice. As shown in Figure 4, the results are relatively consistent regardless of the MC starting point. We average the result and compare both to Millennium ice and the recently released AHA ice:
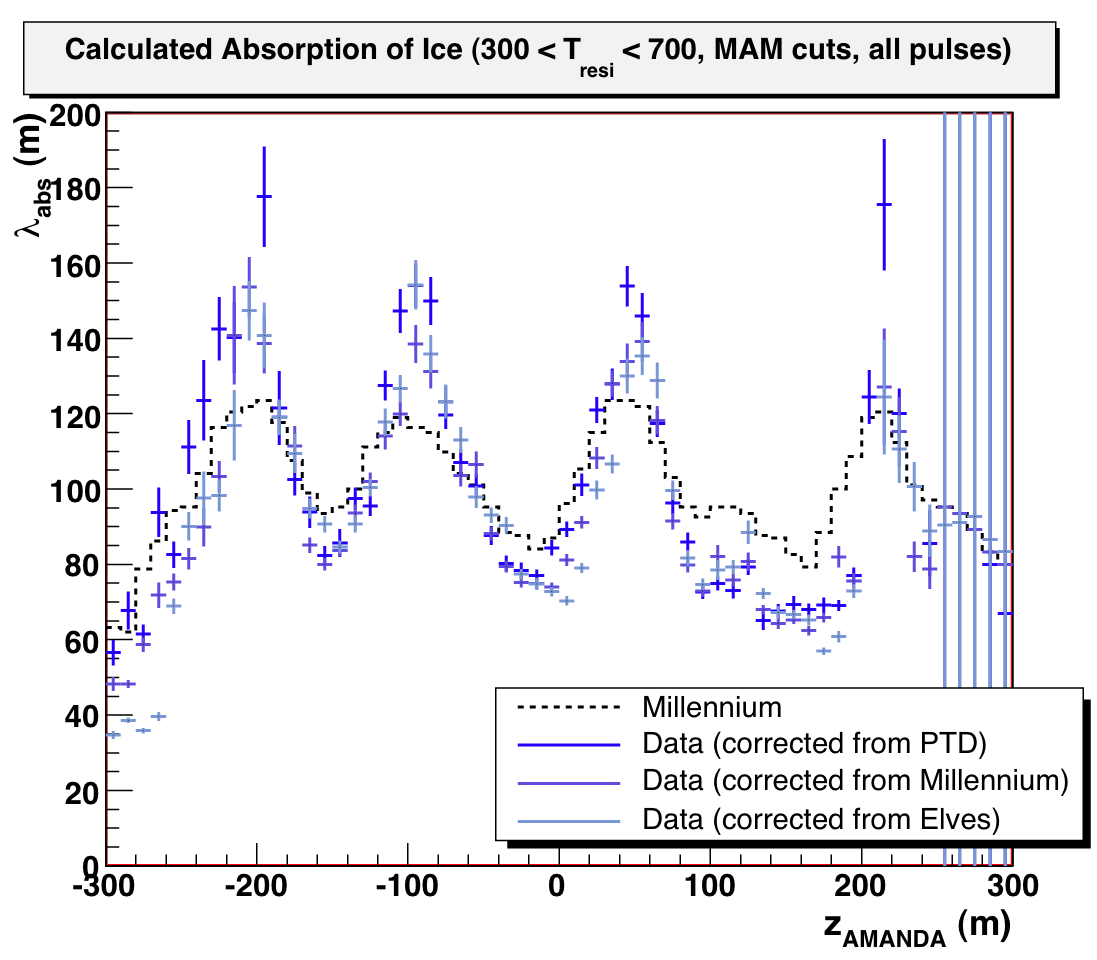 |
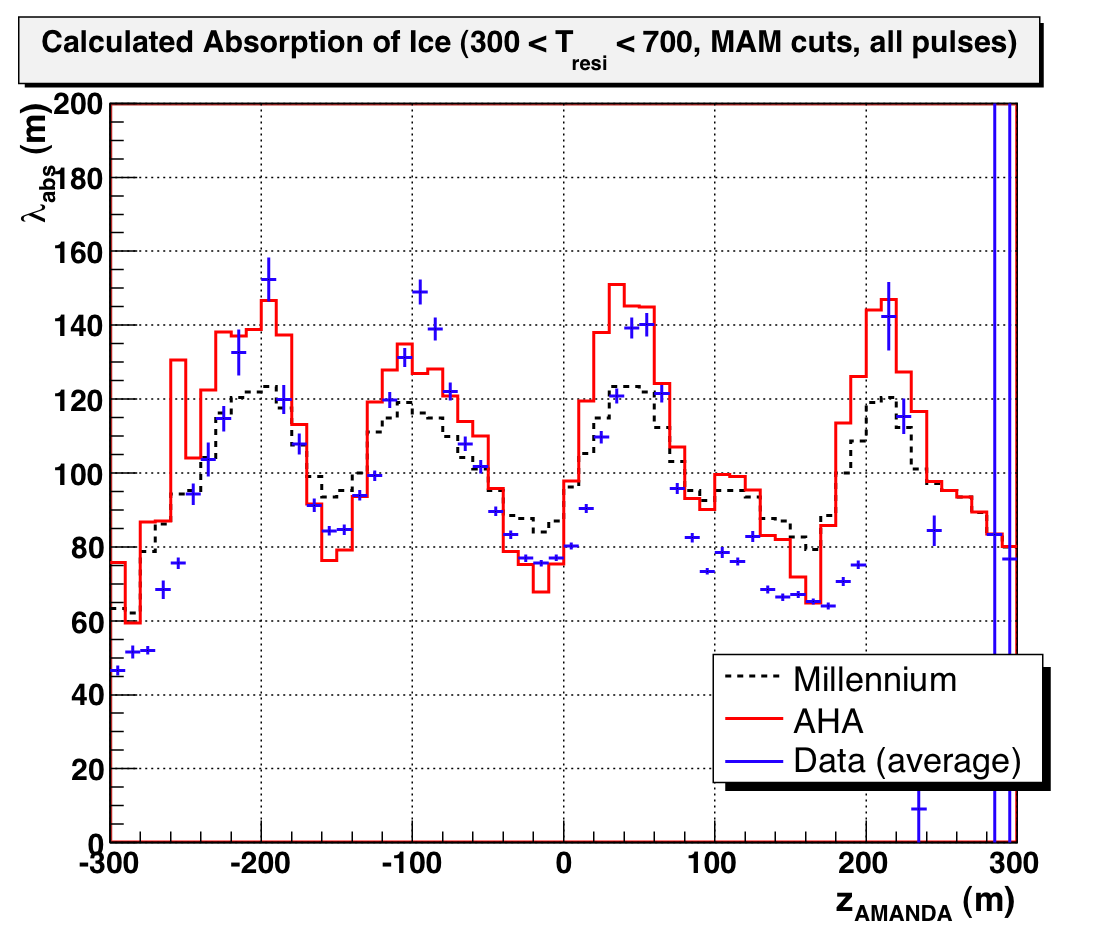 |
Figure 4: Derived peak absorption length. Left: absorption
length as corrected from each of three ice models.
Right: averaged result, compared with Millennium (black) and AHA (red) ice
models.
While there are still some differences between the MAM analysis result and AHA, the scale of the absorption peaks and troughs between the two is much closer than the Millennium model. The main discrepancy is lower overall absorption length in our analysis in the z-ranges from (-300, -250) and (80, 200), although the trough at z=160m matches well. However, there are still possible sources of systematics in this analysis that have not been thoroughly analyzed, such as the effect of OM sensitivity.
This analysis provides independent corroboration that the absorption layers in the Millennium ice model are "smeared out" with respect to the true absorption layers. Furthermore, our results, which were derived without knowledge of the AHA model, agree reasonably well in the magnitude of the correction needed.
Due to continued discrepancies seen between simulation and data in IceCube (see K. Hoshina's page here), we are continuing our investigation into AHA. Looking at lower-level plots, we see that the timing residual slopes do not really agree with the data yet (compare with Figure 1). This is still under investigation.
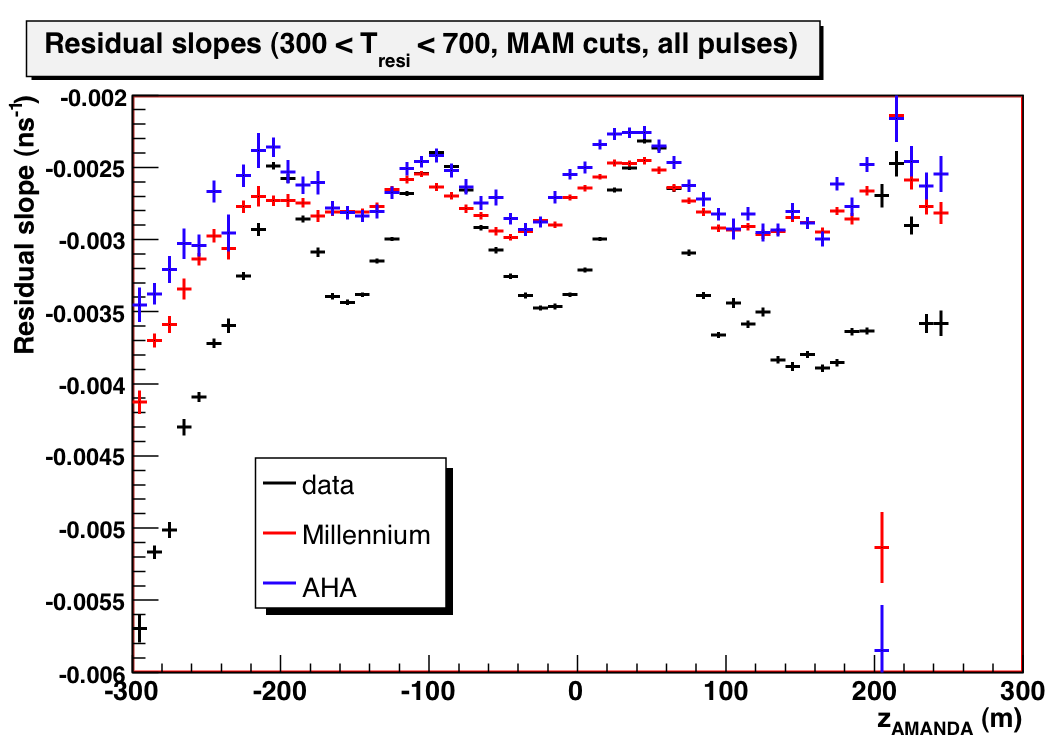
Figure 5: Residual slopes as a function of depth for
data, AHA, and Millennium.
Using this as above to correct the implied absorption length suggests the dust layers are dirtier than modeled:
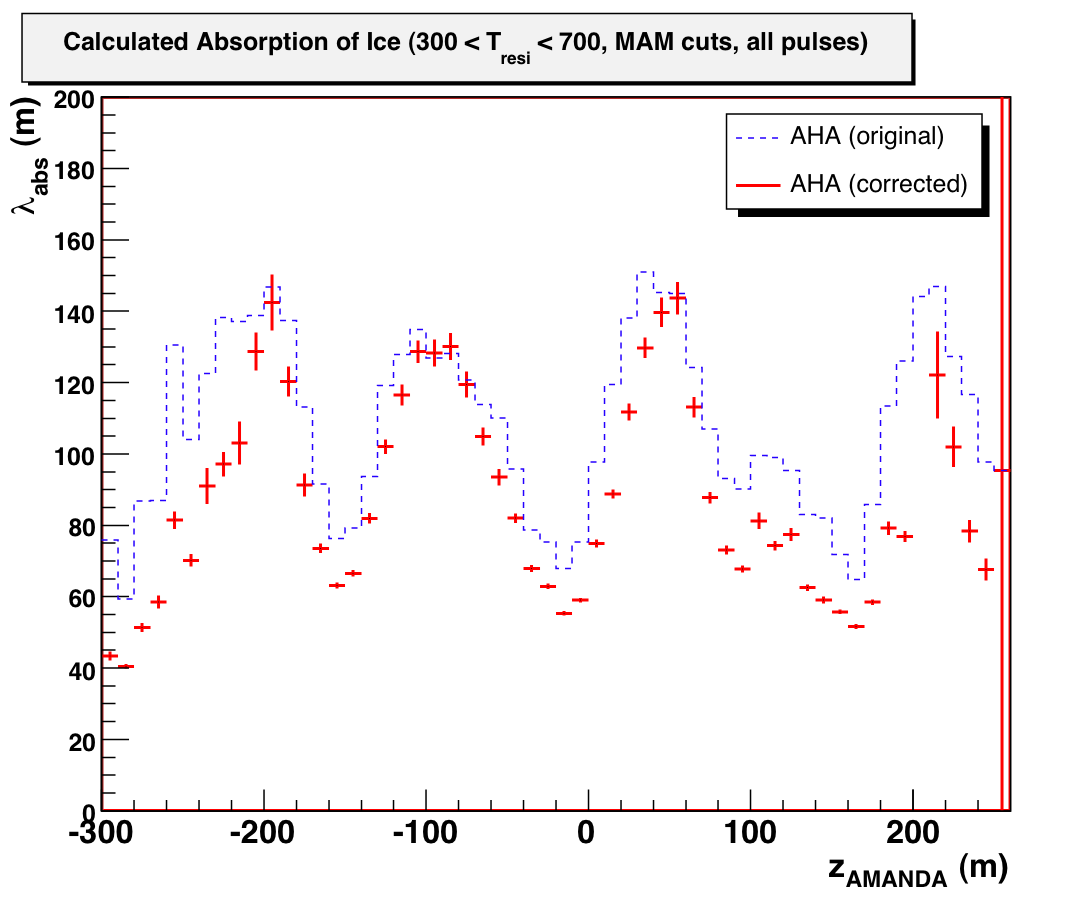
Figure 6: Adjusted AHA absorption length, corrected using residual difference
with data above.