Fitting of atmospheric
neutrino fluxes
I used
a 2-dimensional (log10E, cosJ) fifth-degree polynomial fitting of the original
tables in order to calculate the parameters (15) of the polynomial which can
interpolate the tables. A typical 2-dimensional fifth-degree polynomial is
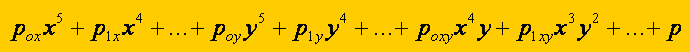
where x = log10E
and y = cosJ.
I did the fitting
by means of the tools provided by ROOT.
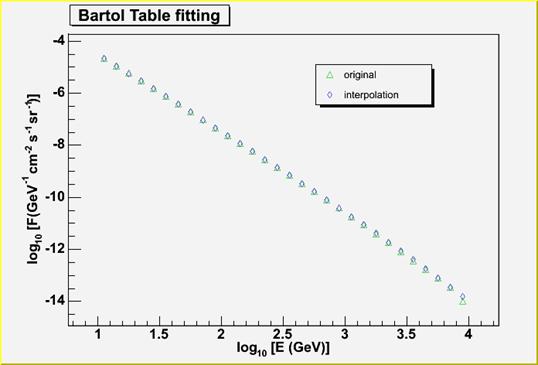
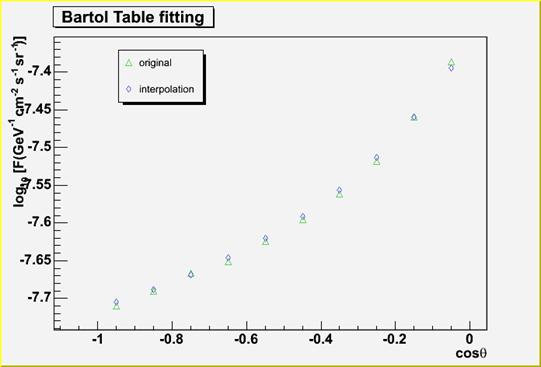
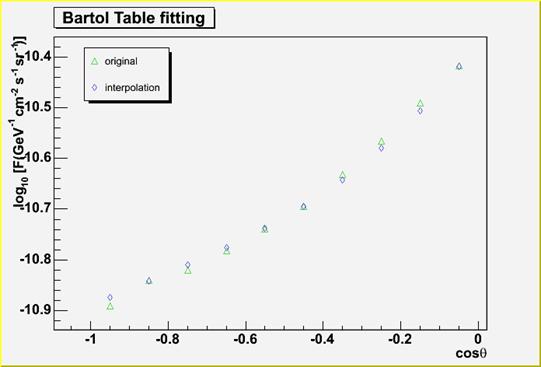
Below, I show some plots of the fitting of the original tables of nm fluxes
(see previous page). More precisely, the
projections X (fixed the cosJ) and Y (fixed the log10E) of the
2-dimensional fitting graph are shown. I consider two plots for each table and
for each projection as example.
Bartol fitting
|
Original table features |
||
|
|
Energy |
cosJ |
|
Binning |
30 |
20 |
|
Range |
10 ÷ 10 4 GeV |
-1.0 ÷ 1.0 |
|
neutrino flavours |
nm anti-nm ne anti-ne |
|

Honda (HKKM 2005) fitting
|
Original table features |
||
|
|
Energy |
cosJ |
|
binning |
101 |
20 |
|
range |
10-1 ÷ 104 GeV |
-1.0 ÷ 1.0 |
|
neutrino flavours |
nm anti-nm ne anti-ne |
|

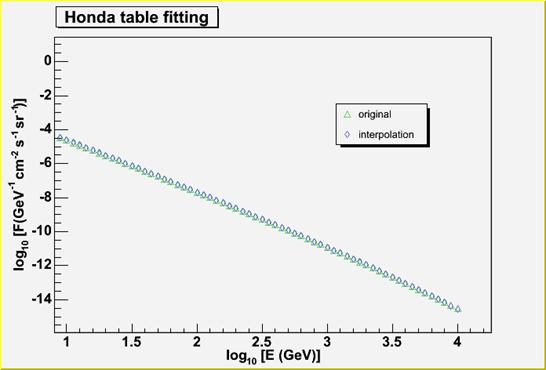
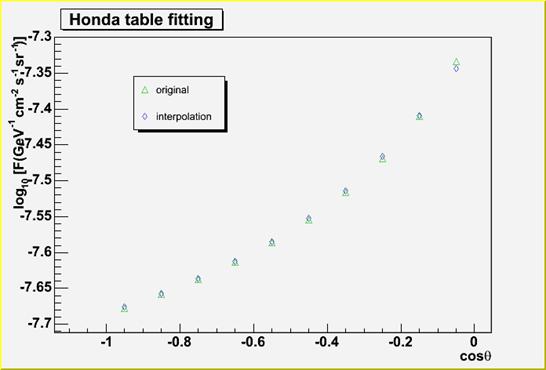

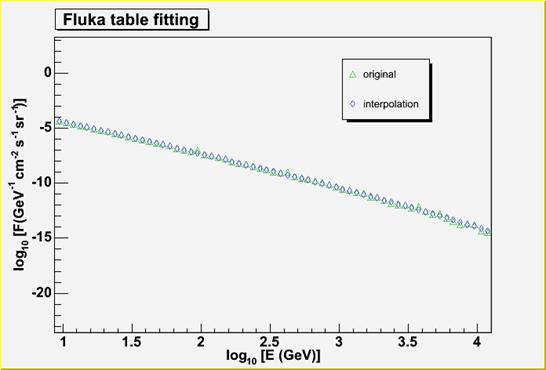
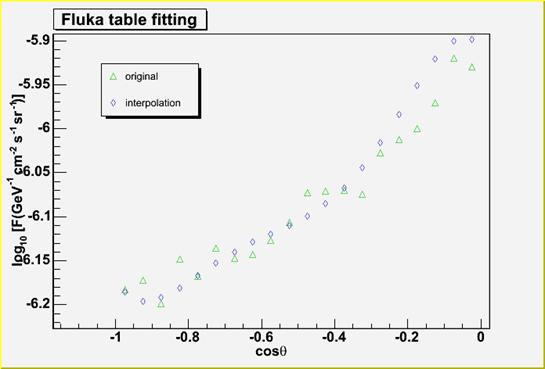
FLUKA fitting
|
Original table features |
||
|
|
energy |
cosJ |
|
binning |
160 |
40 |
|
range |
10-3 ÷ 105 GeV |
-1.0 ÷ 1.0 |
|
neutrino flavours |
nm anti-nm ne anti-ne |
|

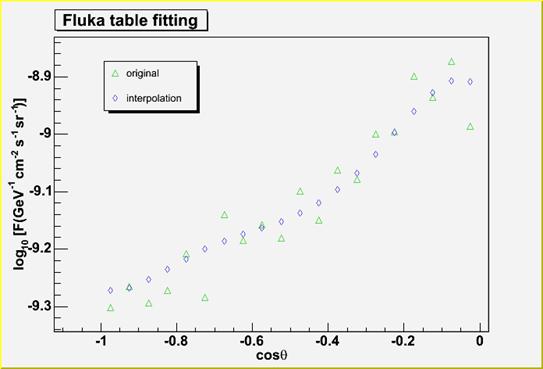
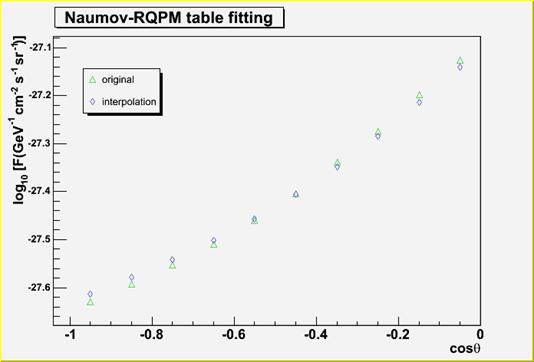
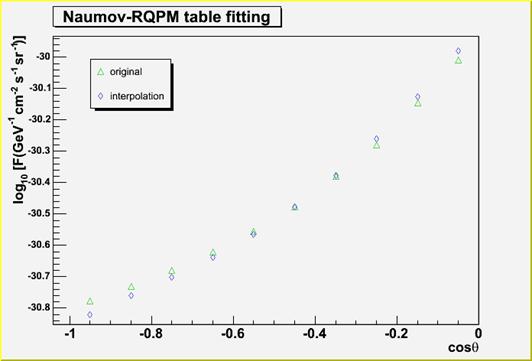
Naumov-RQPM (prompt) fitting
|
Original table features |
||
|
|
energy |
cosJ |
|
binning |
100 |
10 |
|
range |
1 ÷ 109 GeV |
-1.0 ÷ 0.0 |
|
neutrino flavours |
nm anti-nm ne anti-ne |
|


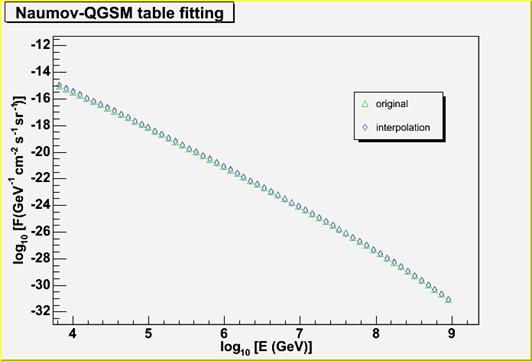
Naumov-QGSM (prompt) fitting
|
Original table features |
||
|
|
energy |
cosJ |
|
binning |
100 |
10 |
|
range |
1 ÷ 109 GeV |
-1.0 ÷ 0.0 |
|
neutrino flavours |
nm anti-nm ne anti-ne |
|
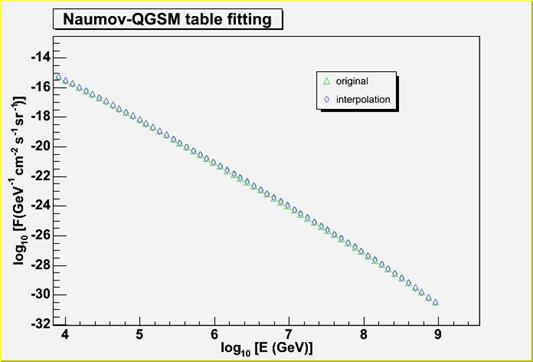
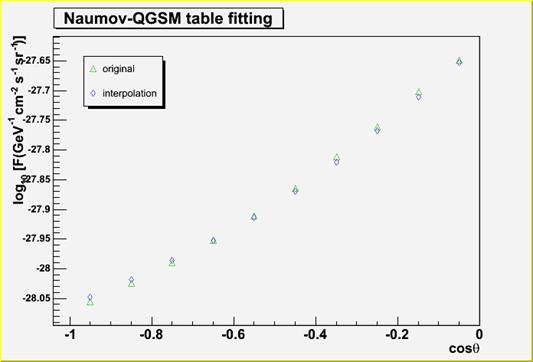
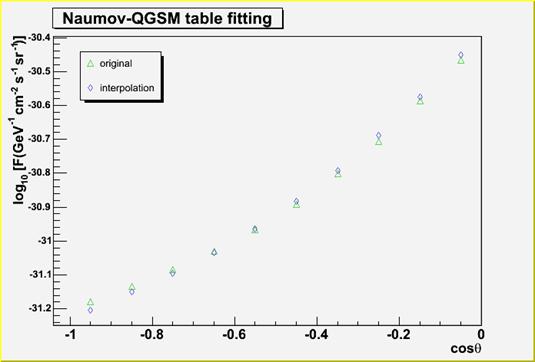
The parameters were chosen so that the c2<<1.
Below I show the range for energy and cosine of zenith
angle used to fit the original tables.
|
Range for energy and cosine
of zenith angle used to obtain the best parameters |
||
|
Original Tables |
Energy range used |
Cosine range used |
|
Bartol |
100.985 ÷ 104.015GeV |
-1.0 ÷ 0.0 |
|
Honda |
100.985 ÷ 104.015GeV |
-1.0 ÷ 0.0 |
|
FLUKA |
100.985 ÷ 104.015GeV |
-1.0 ÷ 0.0 |
|
Naumov-RQPM |
103.975 ÷ 109.025 GeV |
-1.0 ÷ 0.0 |
For values of
cosine in the range 0.0 ÷ 1.0, I considered the same parameters as the
parameters obtained in the range -1.0 ÷ 0.0, because for energy greater than 10
GeV the fluxes for downward-going neutrinos and upward-going neutrinos are
quite the same.