Atmospheric Neutrino Unblinding Proposal
AMANDA-II 2000-2006

John Kelley, UW-Madison, May 2008

5. Simulation

To simulation our atmospheric neutrino prediction, we have generated nine periods of MC (2000p1, 2000p2, 2000p3, and each year from 2001 to 2006) with the following settings:

Generator	nusim
Spectrum	E^-1.5
Zenith range	80-180
Energy range	10 GeV to 100 PeV
Detector MC	Amasim aluminum-opt5
Photon propagation	Photonics 1.54-caustic
Ice	AHAv1, finely binned

Furthermore, to model the systematic effects of OM sensitivity, we have generated each of these nine periods with seven different OM sensitivities (existing sensitivities scaled from 70%-100% in 5% increments), for a total of 63 MC sets. At the final cut level, after reweighting to an atmospheric spectrum we have approximately 60 years of effective livetime for each OM sensitivity.

Observables

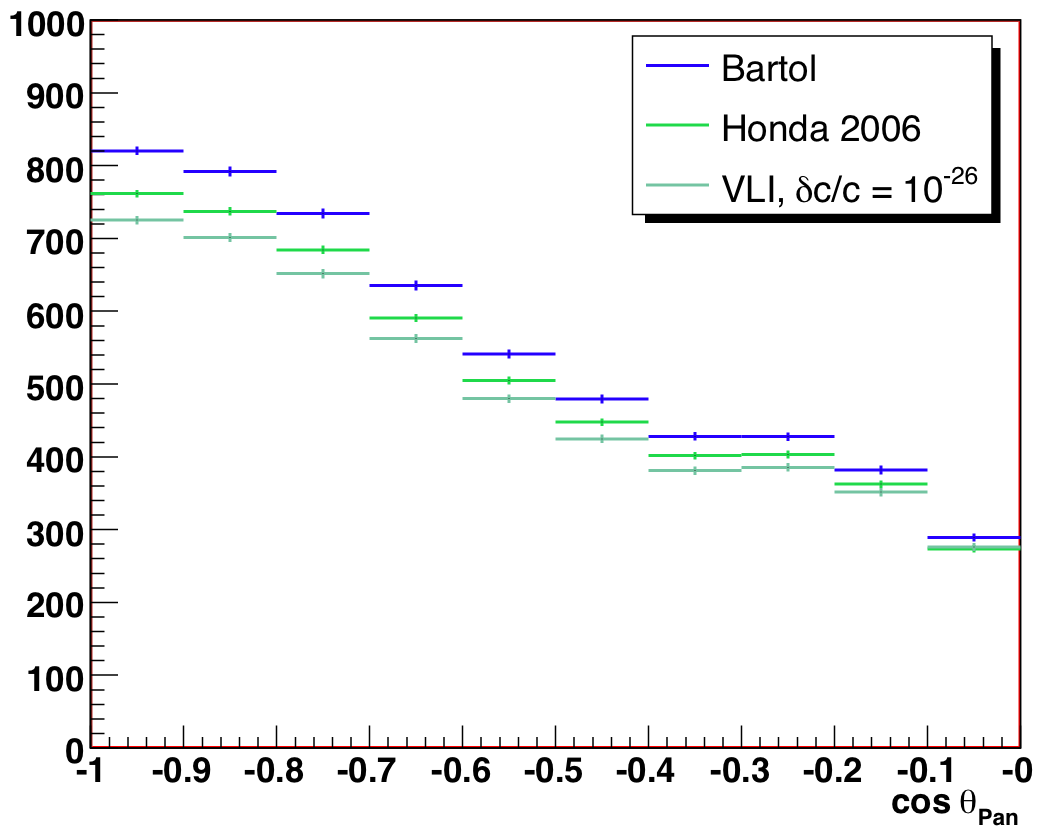
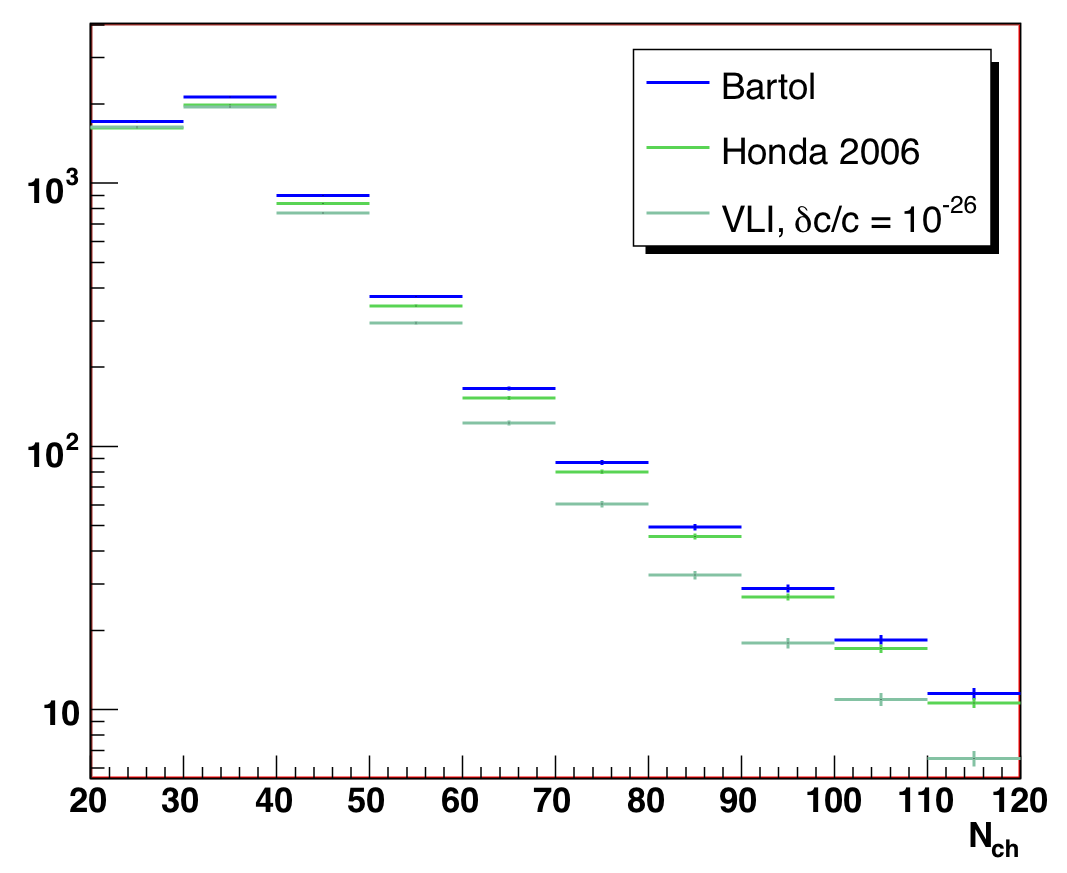
Figures 5.1 and 5.2 below show the two observables, cos(Zenith) and Nch, at the final cut level, and for the binning and ranges suggested in the analysis section, for two conventional atmospheric neutrino models and a moderate value of the VLI parameter delta_c/c of 1e-26.

Figure 5.1: Reconstructed zenith angle, 2000-2006 atmospheric neutrino simulation with final cuts applied, for Honda, Bartol, and Bartol+VLI.

Figure 5.2: Number of channels hit, 2000-2006 atmospheric neutrino simulation with final cuts applied, for Honda, Bartol, and Bartol+VLI.

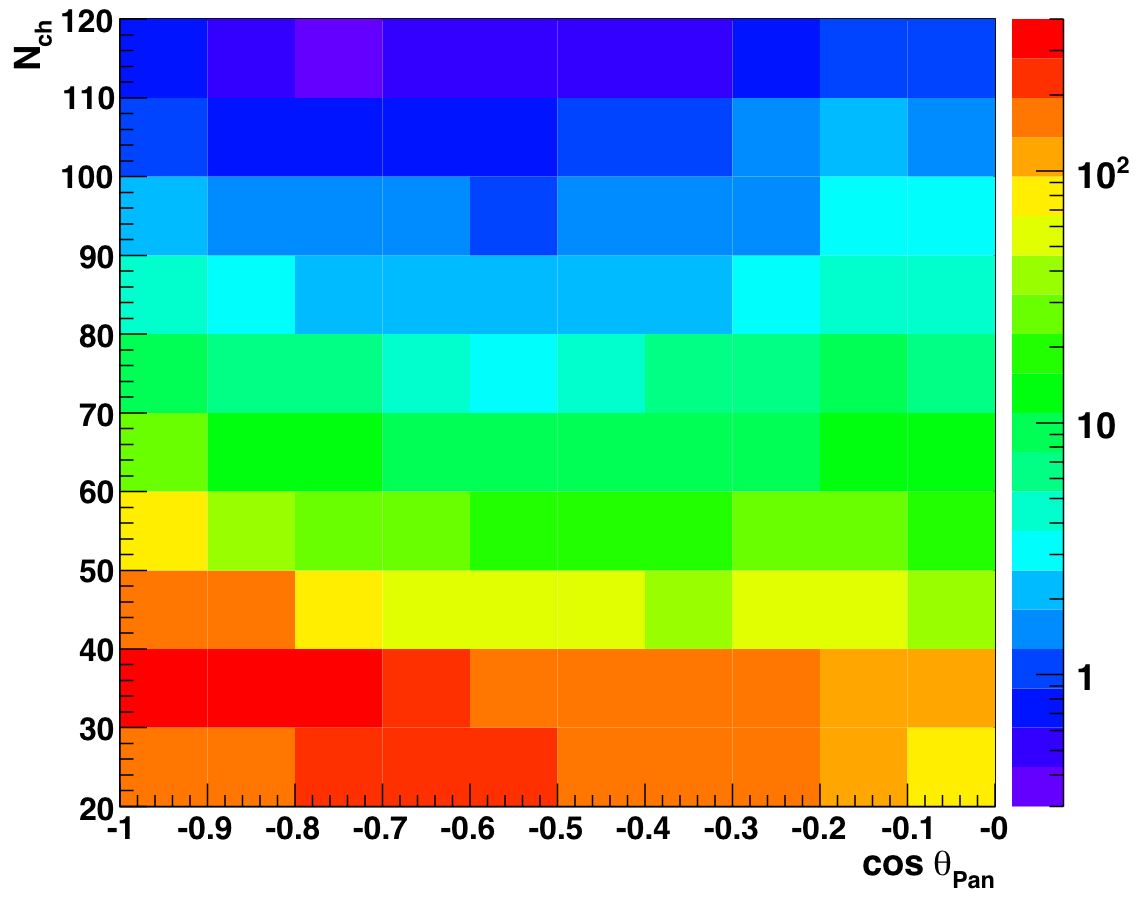
Here we show the same comparison, but in the two-dimensional space in which the likelihood analysis works (the binning and variable ranges are the same). The conventional Bartol flux is on the left, while the VLI oscillations are on the right:

Figure 5.3: Nch vs. cos(Zenith), 2000-2006 atmospheric neutrino simulation (Bartol)

Figure 5.4: Nch vs. cos(Zenith), 2000-2006 atmospheric neutrino simulation (Bartol + VLI)

The distributions are hard to tell apart by eye, but the ratio plot is illuminating, and one can see the effect of the oscillations as we lose muon neutrinos at high Nch and long baselines (close to vertical):

Figure 5.5: Ratio of VLI to conventional flux, Nch vs. cos(Zenith), 2000-2006 atmospheric neutrino simulation

The likelihood analysis can easily tell these cases apart, even with systematic errors applied.

Energy Response

The following figures show the energy response of the detector at the final cut level, as well as the relationship between energy and number of channels hit, using a simulated Bartol atmospheric flux.

Figure 5.6: Simulated atmospheric neutrino energy spectrum at the final cut level. Median energy is 640 GeV; 5%-95% range is 105 GeV to 8.9 TeV.

Figure 5.7: Simulated atmospheric Nch vs. log(Neutrino energy).